Angle Sums and Exterior Angles of Triangles Worksheets
Triangles are one the most interesting geometric shapes there are. They have some unique properties that can be used to help us much better understand exact measures within them and around. This may be why a complete branch of mathematics (trigonometry) is entirely devoted to them. Triangles have three sides and three internal angles. The angle sum theorem states that when you add all those interior (inside) angles of a triangle together, the sum will always measure 180 degrees. You will find that when you advance to writing geometric proofs, this is a commonly used theorem. Exterior angles of a triangle are created by extending the line of an adjacent side. Since this straight line forms a 180 degree angle, to determine the measure of the exterior angle we would subtract 180 by the measure of the interior angle. What is interesting, if you are keeping up with the math, the measure of that exterior angle is also equal to the sum of the two opposite interior angles. This gives us two methods for determining the value of the exterior angle. These worksheets and lessons will help you learn how to determine all these measures.
Aligned Standard: Grade 8 Geometry - 8.G.A.5
- Using References of a Triangle Step-by-Step Lesson- Use the measures of the triangles to determine the missing supplementary angles. Just line them up and find the difference between them.
- Guided Lesson - Use parallel lines, a transversal, and one angle to figure out the measure of a whole bunch of unknowns.
- Guided Lesson Explanation - Once you get the hang of these types of problems, geometry just comes a lot easier to you.
- Independent Practice - These are some really great practice problems for you to tackle.
- Matching Worksheet - One scenario and a whole bunch of missing measures to figure out.
- Transversals Five Pack - Which one is the transversal? There are three lines flapping all about that you need to make sense of.
- Exterior Angle Worksheet Five Pack - A mixed bag of questions and difficulty levels.
- Sum of Interior Worksheet Five Pack - Find the sum of the outside measures in most cases. There are sum problems as well.
- Answer Keys - These are for all the unlocked materials above.
Homework Sheets
A unique set of problems that are all about finding that missing angle.
- Homework 1 - Solve by using the Triangle Angle Sum Theorem.
- Homework 2 - We will first find ∠c. This will help us find d since they should be the same measure. 102 degrees and angle c makes linear pair so their sum is 180 degrees.
- Homework 3 - The line de is drawn parallel to the base bc in the triangle abc. If the ∠a = 15 degrees, find the
Practice Worksheets
This skill has made its way into every single assessment we have seen on this grade level, since the core curriculum was adopted.
- Practice 1 - a and b are two parallel lines with eg and ts as the transversal. Find the value of x.
- Practice 2 - Given: Line f is parallel to line g. ∠1= 111 degrees and ∠4= 43 degrees. Find the measures of ∠2 and ∠3 in the figure.
- Practice 3 - Based on the information provided, determine the values of the angles.
Math Skill Quizzes
Special note: Make sure that students realize that these angles are not drawn to scale.
- Quiz 1 - The lines b and c are parallel. Find a. These are the types of problems that you can expect.
- Quiz 2 - You will find missing measures when given some statements to work off of.
- Quiz 3 - Locate the transversal in the pack of wild lines.
What Is the Angle Sum Property of a Triangle?
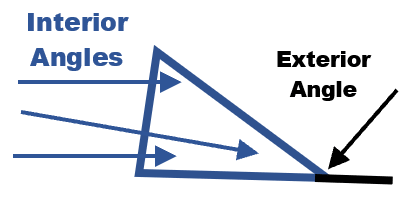
This property simply states that the sum of all three angles within a triangle add up to a total of 180 degrees. Regardless of how a triangle is classified (acute, obtuse, or right) all of those interior angles always equal 180 degrees. This can be very helpful to find the measures of unknown interior angles within a triangle. All you need is the measure of two of the angles and subtract their sum from 180 degrees to determine the missing angle. You will find that this property will be used very often when writing geometric proofs.
How to Find the Exterior Angle of a Triangle
Since triangles have three sides and are closed structures the also have three angles found inside them. These are referred to as interior angles because they are found on the interior of the triangle. The angles that are located outside of the triangle are referred to as exterior angles. These exterior angles have more specific characteristics than just lying on the outside of a triangle. An exterior must linearly pair with an interior angle. It means that an exterior angle must be right next to an interior angle (they share a side), and the exterior and interior angles together form a straight line. You can use this to your advantage by easily forming an exterior angle by extending any one side of the triangle. There can be multiple ways to form an exterior angle. It depends on which side of a triangle you choose to extend. For a triangle, the measure of an exterior angle (d) is equal to the sum of two interiors (a, b) where d = a + b. The exterior angle (d) is greater than two interior angles (a, b). If you know the values of two interior angles, you can easily find the measure of an exterior angle. These worksheets and lessons help students understand how to find the missing measures of angles by using other angles within or outside of the triangle itself.
Why Is It Important to Master These Skills?
Geometry skills like these do not suggest to many students that they are that vital to everyday life and for the most part that is true. There are many situations in real life where these skills are not only vital, but paramount to be able to complete the project and life and death, when you consider safety. The Angle Sum Theorem allows architects to design structures that are free floating. Any building that is not a rectangle or square from top to bottom has this theorem to thank, not only for the construction, but also for the ability to create and fulfillment of a safe structure or dwelling that people can inhabit. This is not only true of building and homes, but just about any physical design you are making. Almost all electronics today run off of a chip set of some kind. The housing that encases them needs to sturdy and secure. Otherwise, every time you slightly rattled this device it would stop working. These housing that encase the chipsets are all designed with this Theorem in mind. The next time you drop your phone and it still works, you know who to thank.